The discovery of an unusually massive neutron star with a white dwarf companion was revealed in a paper published by John Antoniadis, a PhD student at the Max Planck Institute for Radio Astronomy and others on the international team this past April. Using radio telescopes from observatories around the planet to identify and study the neutron star, and the European Southern Observatory’s (ESO) Very Large Telescope (VLT) with its FORS2 spectrograph located at the Cerro Paranal observatory in Chili, to study the white dwarf star, the astronomers have discovered the most massive neutron star found to date. Labeled as PSR J0348+0432, the neutron star weighs in at twice the mass of the Sun.
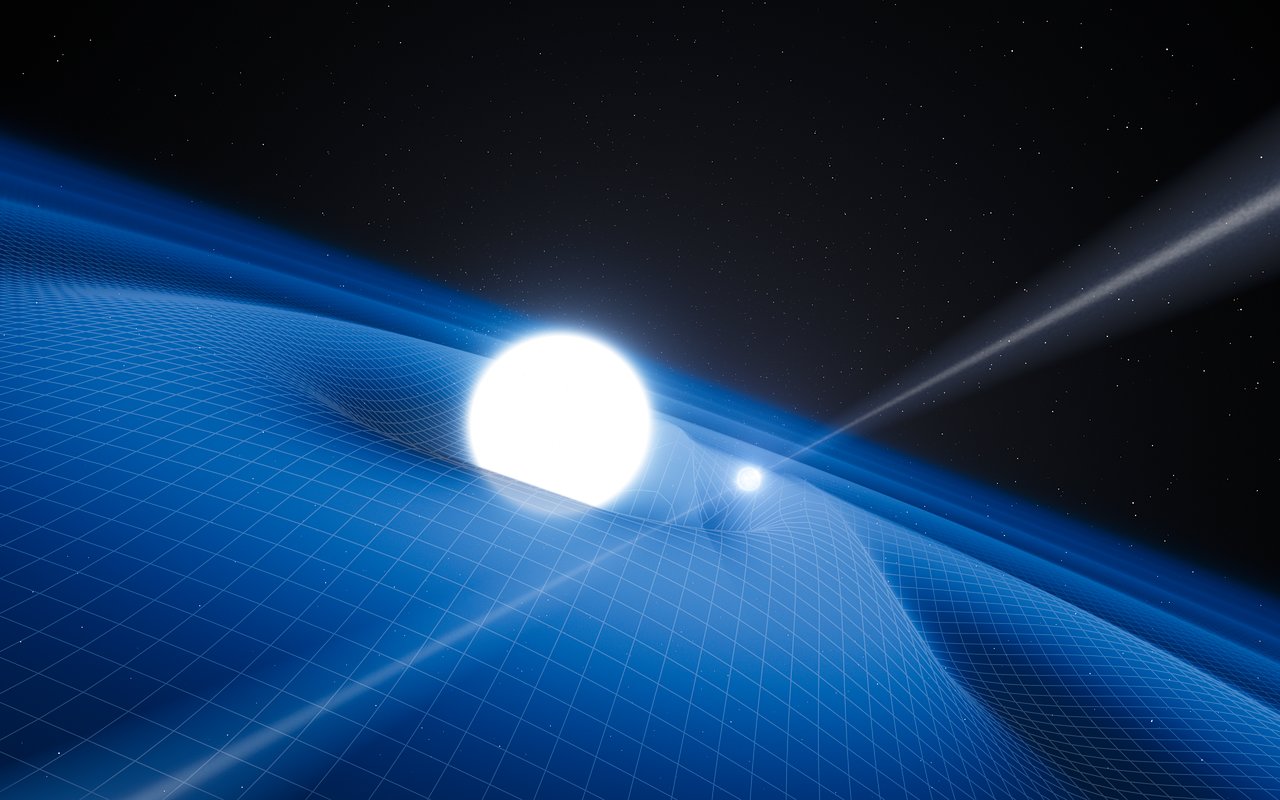
White dwarf star orbiting a pulsar, a neutron star beaming radio frequency energy, generating gravity waves as they revolve about a common center. Image courtesy of ESO
So what? One might ask.
Well, what’s remarkable is that this mass exits in a sphere only 12.4 miles (20 km) in diameter. This means that the density of the material inside this defunct star is on the order of 1 billion tons per cubic centimeter—the size of a sugar cube! The force of gravity on the surface of the star is 300 billion times stronger than what we experience here on Earth.
This super dense ball is rotating 25 times per second and has a white dwarf companion star with a mass 0.17 that of our Sun and a diameter of 56,000 miles (90,000 km) orbiting it every 2.5 hours. This neutron star is also a pulsar. It sends a highly directional beam of radio frequency energy out into the cosmos and provided the pulsating beacon that we detected to locate this unique stellar system.
This super massive star along with it’s companion provides a wonderful natural laboratory for Earth based astronomers to study Albert Einstein’s General Theory of Relativity, which describes how space is curved by mass and energy and we observe in part as gravity. Studying this high intensity gravitational system may help us better understand gravity waves, predicted by Einstein’s theory, and explore the realm where general relativity and quantum mechanics may meet.
The team of astronomers have already measured a reduction in the orbital period of 8 millionths of a second per year due to energy being radiated from the system by gravity waves, as predicted by general relativity. Although gravity waves have been inferred by this and other binary systems, they have yet to be detected by the Laser Interferometer Gravitational Wave Observatories—LIGO—facilities on Earth.
But, wait! A sugar cube size piece of neutron star stuff that weighs 1 billion tons? How do you wrap your head around that piece of information? How do you compare that to everything you touch in your day-to-day routine? Let’s see what a billion tons of stuff might look like.
A good, massive object that most people have a concept of might be the African bull elephant.
Weighing in at about six tons on average, ten feet high by twenty feet long and eight feet wide, we would need only 167 million bull elephants to equal one cubic centimeter of neutron star material. That’s a lot of elephants!
To get a better perspective on how large this number of elephants is, consider if you packed these pachyderms side by side, front to back, you would cover an area of 26.7 billion square feet. (Whoops! We’re back to billions again. Better to convert that to square  miles/kilometers.) That’s 956.5 square miles (2477 sq km); equivalent to a square with sides 30.93 miles (49.8 km) long. You could comfortably park them all in the tiny country of Luxemburg, which has an area of 998 square miles (2586 sq km), with a little room to spare.
miles/kilometers.) That’s 956.5 square miles (2477 sq km); equivalent to a square with sides 30.93 miles (49.8 km) long. You could comfortably park them all in the tiny country of Luxemburg, which has an area of 998 square miles (2586 sq km), with a little room to spare.

How about something bigger, even more iconic, like the Empire State Building (ESB). Standing 1,454 ft (443.2 m) high, it has an estimated weight of 365,000 tons. We would need only 2740 ESBs to offset a balance with 1 sugar cube-size piece of neutron star stuff on it. That’s at least a number we can begin to have an intuitive sense for.
So how much area would 2740 ESBs cover? With a foot print of 79,288 ft2 (7240 m2 ) or .003 square miles (.007 square km), our collection of buildings would cover 7.8 square miles (20.3 sq km) – about 1/3 of the island of Manhattan, which has an area of 22.96 square miles (59.5 sq km). It’s a bit hard to imagine a third of Manhattan covered in Empire State Buildings. But, we can reduce the number and get a better handle on a billion tons.
Let’s take our Empire State Building and make it completely out of gold, all 37 million cubic feet (1.04 million cubic meters) of it. With gold weighing 1204 pounds per cubic foot, the solid gold building would weigh 44.5 billion pounds or 22.3 million tons. Now all we would need is 45 of these precious metal buildings to reach 1 billion tons.
This gilded collection would cover about 23 city blocks or an area from where the ESB is now to Times Square, assuming two buildings per block. Try to imagine this the next time you fly to New York City: the core of downtown Manhattan populated with 45 gleaming, solid gold Empire State Buildings and all that is equivalent to 1 cubic centimeter—one sugar cube-size of neutron star stuff.
Hopefully this helped you get a little better feel for what a billion tons might be. It’s helpful to do these simple calculations and comparisons and try to put into perspective or get a better grasp on some of the enormous numbers that come out of the study of this amazing Universe we live in.
When considering the cosmos and all the numbers we produce to describe it, I cannot help but feel that all we hold dear on this tiny blue planet, floating through the vastness of space, is insignificant when compared to what we are immersed in. Yet, we are sentient beings, and curious about the Universe we live in and that makes us very significant, because for all we know now, we are the only creatures in this entire cosmos that are looking up and asking these big questions.
Till next time,
RC Davison
Link to the research paper: “A Massive Pulsar in a Compact Relativistic Orbit”, by John Antoniadis et al.

